Web Design by US!!!    all rights reserved 2002

Experiment --> 1 2 3 4 5 6 7 8 9

Experiment 5

    A light clock lies on its side on a railroad car as the car moves to the left with velocity v.

Question: How is the length of the clock affected?

Answer:

We know that the time between ticks on the moving clock, as we see it, will be t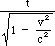´ = . Let,

t₁ = time that takes the light to go from the first mirror to the second , as we perceive it.
t₂ = time that it takes the light to go from the second mirror back to the first, as we perceive it.
L´= length of the light clock, as we perceive it.

We can analyze the situation as follows:

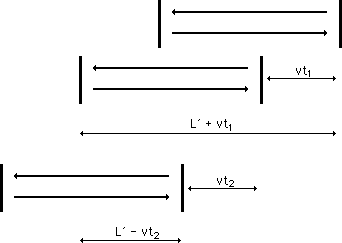
From the above picture we see that the distance the light traveled in time t₁ was L´+ vt₁. However, this distance is also equal to ct₁ (rate x time). Also, the distance the light traveled on the return trip was L´- vt₂ = ct₂. Solving for t₁, we have

L´+ vt₁ = ct₁

which implies that L´= ct₁ - vt₁,

which implies L´= (c - v)t₁,

which implies t₁ = .

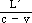Similarly,

L´- vt₂ = ct₂

which implies that L´= ct₂ + vt₂,

which implies L´= (c + v)t₂,

which implies t₂ = .

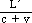Thus,

t´ = t₁ + t₂


=


=


=


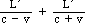
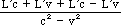
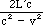
which implies that = .

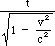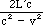Since t = (2L)/c,

=


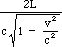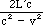
which implies that = ,


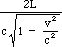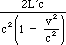which implies = ,


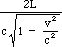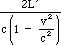which implies = ,


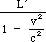which implies L´ = L .

Conclusion: 

    Since is less than 1, this shows that we will measure the length of his light clock as being less than ours. Thus, when an object is moving in a straight line with a fixed velocity v, we will see its length, as measured in the direction in which it is moving, shorten.